Valve acceleration, acceleration valve, acceleration valve gear train, calculate cam lobe profile, calculate cam shaft, optimize cam lobe design, cam lobe profile

Our cam calculation secret to success is in the background, invisible
www.gaenssle.de
30% more valve lift while reducing forces by 60%
How do we do that?
Read on - we will reveal the secret.

All you need to succeed: correctly calculated cam lobes!

A preliminary note:
Calculating the cam of a combustion engine can be done for redesign, replacement or improvement. Let us demonstrate the power of calculating cam lobes with KnockSoft using a typical example which is in no way extraordinary. Comparing the original cam lobes and the newly calculated lobes is the best way to show the possible improvement.

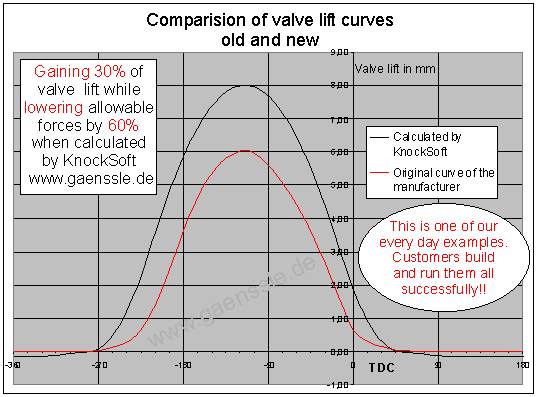
Valve lift curve
The valve lift curve shows the lift of a valve over the crankshaft rotation. 0 degree crankshaft rotation equals top dead center (TDC) of the piston. The cam timing displays during how many degrees of crankshaft rotation the valve is opened. Usually (like in this example) the valve timing is measured at 1mm valve lift, i.e. the intersection point of a line parallel to the x axis at 1mm lift and the valve lift curve.

The above diagram shows the valve lift curves of a four stroke engine. Red - factory stock, black - as calculated by KnockSoft. At the first glance two valve lift curves without extremal values. The black curve has a duration of 255 degrees, the red one has less lift and duration. There is nothing especially noticeable, even for those who already have measured, analyzed or otherwise have dealt with such curves.

The extremely serious advantages of KnockSoft cam lobes are invisible when looking at the camshaft itself or at the valve lift curves. But analyzing the actual acceleration shows the huge advantages of cam lobes calculated by KnockSoft. (See the diagram below). The force necessary for moving the valves grow proportionally with acceleration and moving mass:
Newton's law: F = m x a
Force equals mass by acceleration
Mass and acceleration are the indicators for the dynamic load capacity and the quality of the valve train.
Low mass and low acceleration mean low forces necessary to operate the valves. They stand for a high rev, durable and low friction valve train. In other words: There is only a limited time available for opening and closing the valve. The cam lobe should be calculated in a way to ensure that process is taking place just in a controlled manner at maximum engine speed along with lowest forces necessary.

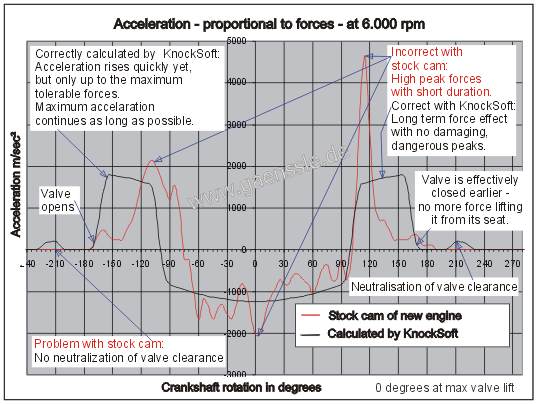
Before opening the valve:
The valve, as accelerated by KnockSoft - black curve - experiences a soft elimination of the valve clearance just before opening starts, in order to minimize possible oszillation. The red curve doesn't do so, or much too late. The opening can be superposed by oszillations.

Opening the valve:
The black curve runs up the acceleration as quickly as possible, yet only up to a computable, acceptable limit. Exceeding these limits may cause bent pushrods or valves, seizures or premature wear. Acceptable limits can be computed exactly. An acceptable limit may be e.g. the bending force of a pushrod, calculated by Euler's formula. The surface pressure between cam lobe and flat-base tappets may be such a computable limit. The red curve wastes valuable opening time with no important acceleration, while "catching up" later with two unneccessary high acceleration peaks. Anything accelerated for a long time reaches high speed - something we know when driving a car or from physics lessons. Therefore, the black curve stays at maximum acceleration as long as possible.

Transit to max lift and starting to return to the valve seat:
At the end of the opening acceleration the black curve changes its sign immediately. The valve is decelerated as quickly as possible by the valve spring and stands still for a short period of time at maximum lift. The red curve procrastinates when changing its sign, again wasting valuable time. The red curve shows several peaks of up to 30% over the required value, both at deceleration until max lift as well as at the acceleration for returning to the valve seat.

Closing the valve:
The valve is accelerated back to the valve seat by the valve spring. Prior to thudding onto the seat, the valve must be "caught" smoothly, i.e. decelerated. Both acceleration curves change sign again and rise into positive values. The red curve does that not as hesitantly as at the first sign change, but it exceeds the black curve's value by 250% (5,700 m/sec²). After the valve has been decelerated with unnecessarily high forces, the remaining time is wasted with acceleration far beyond the acceptable maximum. At the end of the closing process, with the black curve the valve sits on its seat under full contact pressure and transfers its heat to the cooling seat. With the red curve, there are still forces applied. The extended heat transfer cools down the valve which in turn increases power output and decreases emissions. There is no clearly defined elimination of valve clearance with the red curve.

Final note:
It is obviously that the improvement by calculating the cam lobe as described above is not always possible. Once a cam lobe has been computed correctly, there is no gain in re-computing it again.
Important: This calculation is a physics problem, and its solution is either right or wrong.
All solutions containing well known buzzwords as "re-calculated valve lift curve", "modified lobe", "reground cam", "modified center lobe" or "proven cam profile from another engine" have one thing in common - they are wrong.
There is a remarkable number of camshafts which can be improved. Even a 2004 model does not stand for a good camshaft - there are both correct camshafts but also those which are even worse than described above. Camshafts prior to 1980 could be improved with no exception.

Interested?
Order your analysis at Gaenssle as a first step.
We will show you in print whether your camshaft is correct or not.
We have the perfect solution for incorrect cams or new design.